Mathematics Study Guide for the TEAS

General Information

The Mathematics section of the ATI TEAS 7contains 34 scored questions and four that are not scored. You will not know which questions are scored and you will have 57 minutes to answer all of them.

The questions will focus on concepts that pertain to these two main mathematical areas:

• algebra and numbers
• data and measurement

There are only a few more questions that pertain to numbers and algebra than there are concerning measurement and data. This is a significant departure from the previous edition of the TEAS, where only about one-fourth of the math questions were about measurement and data.

Types of Questions

Some of the questions in each section of the online version of the TEAS 7 are presented in slightly different formats. Instead of all typical multiple-choice questions with four answer choices, you will find a few structured in a new way. Please check out our explanation of these question types so you’ll be prepared. Scroll to the second heading on the linked page.

Numbers: Part 1

About half of the questions on the TEAS 7 deal with basic number concepts, such as the four basic operations and how numbers work when they are applied. The word problems related to this area of math will also require you to know when and how to use these operations. The numbers dealt with in number questions include positive and negative whole numbers, as well as fractions, percentages, and decimals. Be sure you are familiar with all these concepts and can manipulate all the number types accurately using each operation.

Fractional Parts of Numbers

Fractions pop up often in life:

• Your car’s gas gauge shows fractions of a tank.
• English system rulers are divided down to sixteenths of an inch.
• Stores have half-off sales.
• Recipes often have ingredients in fractions of a cup, teaspoon, or tablespoon.

Fractions can also be expressed as decimals or percentages, which we will take a brief look at in this section.

Fractions

In mathematics, fractions represent equal parts of a whole thing or group. The bottom number, called the denominator, represents the total number of equal parts making up the corresponding whole. The top number, called the numerator, indicates how many of the total equal parts there are. The numerator and denominator are separated by a line called a fraction bar.

\[\frac{\text{numerator}}{\text{denominator}}\]

Mixed Numbers

The term “mixed number” refers to a number that includes a whole number and a fraction, such as \(3 \frac{1}{8}\) or \(97 \frac {5}{16}\).

Improper Fractions

An improper fraction is one whose numerator is larger than its denominator, such as \(\frac{5}{4}\) or \(\frac{13}{2}\). Most tests require you to change improper fractions into mixed numbers. To do so, divide the numerator by the denominator, producing the whole number and using any remainder as the numerator of the fraction.

\(\frac{5}{4}\) becomes \(1 \frac{1}{4}\).

\(\frac{13}{2}\) becomes \(6 \frac{1}{2}\).

Percentages

A percentage is an amount expressed as part of 100 and is often designated by the \(\%\) sign. To find the percentage of a value, multiply it by the percentage’s decimal equivalent, which is obtained by moving the decimal point two places to the left and dropping the percent sign. For example:

\(60\%\) of \(160 = 0.60 \cdot 160 = 96\)

Decimals

Decimals are numbers that have fractional parts separated from whole parts by a decimal point. Hence, they express values that fall between whole numbers. Here are some examples:

\(0.013\) (read “\(13\) thousandths”)

\(28.04\) (read “\(28\) and \(4\) hundredths”)

\(0.5\) (read “\(5\) tenths”)

Numerical values are written using one or more digits. There are a total of ten digits: \(0,\, 1,\, 2,\, 3,\, 4,\, 5,\, 6,\, 7,\, 8,\) and \(9\). The reason an infinite range of values can be represented, using so few symbols, is that the quantity represented by a given digit changes, depending on its placement within a number. Hence, each position is given its own name, such as tens, hundreds, hundred-thousandths, etc. This is known as “place value.”

A dot, called a decimal point, separates digits that represent whole units from digits representing partial units. Whole units, more commonly referred to as whole numbers, fall to the left of the decimal point, whereas partial units are located to the right. Values that include partial units are commonly referred to as “decimal numbers.”

Here are the first place values on both sides of the decimal in a number:

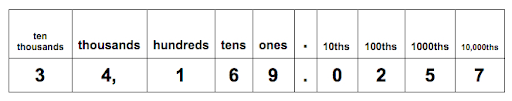
So, this number would be read, “thirty-four thousand, one hundred sixty-nine and \(257\) ten thousandths.”

Converting Fractions to Decimals and Percentages

To convert a fraction to a decimal, divide the numerator by the denominator.

If the problem reads, “Write \(\frac{3}{4}\) as a decimal,” divide \(3\) by \(4\). The answer is \(0.75\).

Converting Decimals to Fractions and Percentages

A decimal can be converted into a fraction by doing two things. First, move the decimal point as far right as possible, counting the places moved. Second, write the resulting number as a numerator and for a denominator write a \(1\) followed by as many zeros as you moved the decimal point.

For example, start with \(3.19\).

• Move the decimal point two places to the right, giving you \(319\).
• Put \(319\) over a \(1\) with two zeros: \(\frac{319}{100}\).
• This could be rewritten as \(3\frac{19}{100}\).

To convert a decimal into a percent, simply move its decimal point two places to the right and follow the last number with a percent sign. Hence, \(0.341\), written as a percent, is \(34.1\%\).

Converting Percentages to Fractions and Decimals

Percent means per hundred. To convert a percent to a fraction, write it over \(100\) and reduce it if possible.

Example:

\[52\% = \frac{52}{100} = \frac{13}{25}\]

If the percent has a decimal part when you write the fraction, multiply its top and bottom by \(\frac{10}{10}, \frac{100}{100},\) or \(\frac{1\text{,}000}{1\text{,}000}\), whatever it takes to clear the decimal. Then simplify, if possible.

Example:

\[3.8\% = \frac{3.8}{100} = \frac{3.8}{100} \times \frac{10}{10} = \frac{38}{1\text{,}000}\]