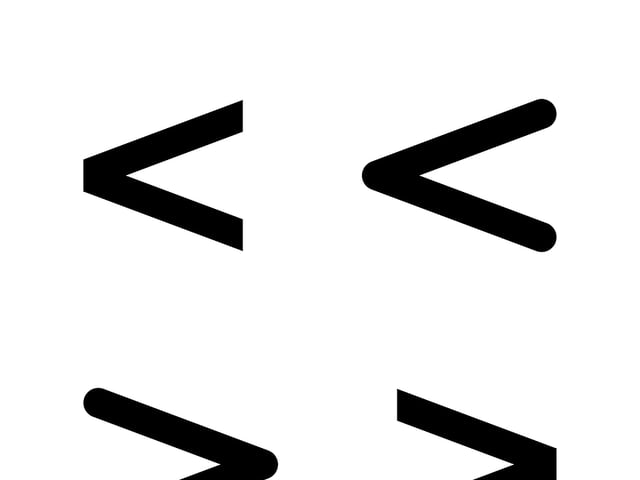
The Greater Than Sign vs The Less Than Sign: How to Remember the Difference

Math symbols are not just a collection of squiggles on a page. Each one holds an important meaning, like a little piece of code in a grand puzzle. Today we’re going to delve into two crucial mathematical symbols: the greater than sign (>) and the less than sign (<). Knowing these signs and distinguishing between them is vital for dealing with equations, making comparisons, and even making sense of real-world scenarios.

Understanding the Signs

The greater than and less than signs are fundamental elements of mathematics, used for comparisons and to describe relationships between different quantities. To master their usage, it’s essential first to understand what each symbol represents.

The Greater Than Sign (>)

The greater than symbol (>) signifies a relational inequality where the value to the left is larger or more than the value on the right. This sign visually leans towards the smaller number, with its open side facing the larger number. It’s as if it’s pointing out the imbalance and emphasizing that one side carries more weight.

Let’s illustrate this with an example. Consider the mathematical statement 7 > 2. Here, seven is on the left side of the symbol, and two is on the right. The greater than sign (>) is indicating that seven is greater than two. In other words, if you were to compare seven and two, seven would be the larger value.

Symbol	Meaning	Example	Description
>	Greater Than	7 > 2	Seven

The Less Than Sign (<)

The less than sign (<), on the other hand, suggests a situation where the quantity to the left is smaller or less than the value on the right. Like its counterpart, the less than sign also leans towards the smaller number, with its wider part facing the larger number.

To illustrate, take the mathematical statement 4 < 9. The number four is on the left of the symbol, and nine is on the right. The less than sign (<) in the middle tells us that four is less than nine. Thus, when compared, nine holds the larger value.

Symbol	Meaning	Example	Description
<	Less Than	4 < 9	Four is less than nine

These signs are more than just symbols; they are a mathematician’s language, helping to describe relationships and make comparisons. By understanding the meaning behind these symbols, we unlock the ability to read and write in this universal language of numbers.

Tricks for Remembering the Difference Between the Greater Than and Less Than Symbols

The Alligator Method

The Alligator Method serves as an excellent way to remember the difference between the greater than and less than signs. It’s particularly helpful for younger learners who benefit from a more visual and playful approach to understanding mathematical concepts.

Imagine the two signs, the greater than (>) and less than (<), as the open mouths of alligators. As we all know, alligators are always hungry and prefer a larger meal. So, the alligator’s mouth will open towards the larger number, eager to gobble it up.

For example, let’s consider the equation 5 > 3. In this case, visualize the ‘>’ sign as the alligator’s mouth. Given the choice between the numbers five and three, the alligator opts for the larger meal—five. Therefore, it opens its mouth towards five. This visual metaphor aids in remembering that the open side of the ‘>’ sign always faces the larger number.

This method, along with a lively imagination, can turn an abstract concept into a concrete and memorable image, fostering a deeper understanding of the greater than and less than signs.

The L Method

While the Alligator Method caters to visual and imaginative learners, the L Method leans more towards linguistic and logical learners. It takes a different approach, transforming the abstract symbols into something more concrete and linguistic.

To utilize the L method, one has to rotate the less than sign (<) 90 degrees clockwise. When you do this, you’ll notice it resembles an ‘L’. This ‘L’ is a reminder of the term ‘Less than’, conveniently starting with the same letter.

For instance, if you come across the inequality 4 < 7, you would rotate the ‘<’ symbol mentally, recognize the ‘L’ shape, and remember that it signifies ‘Less than’. So, four is less than seven.

The L Method offers a linguistic memory aid that can be more effective for those who prefer words and letters over visual imagery. By making a simple mental rotation, you can reinforce the meaning of the less than sign in your memory.

Both the Alligator and the L Methods offer unique approaches to understanding and remembering the difference between the greater than and less than signs. Each caters to different learning styles, ensuring everyone can find a method that resonates with them. Whichever method you choose, regular practice will help cement these symbols in your memory.

Remembering with Real-World Scenarios

One of the most effective ways to understand and remember mathematical concepts is by connecting them with real-world scenarios. These associations make the abstract concepts more concrete and relatable, making it easier to remember their meanings and applications.

Everyday Comparisons

Think about the daily comparisons you make. For example, let’s say you have $10 in your pocket and come across a book you’d like to buy, but it costs $15. In this case, your money is less than the price of the book, which can be represented as 10 < 15. Or, suppose you’re waiting for a train that leaves at 3 PM, but the time now is 4 PM. This means the current time is greater than the train’s departure time, expressed as 4 > 3.

In these examples, the use of the less than and greater than signs provides an accurate comparison between two quantities. By practicing such comparisons, you can get comfortable with these signs and understand their real-life implications.

Practice with Examples

To get the hang of using the greater than and less than signs, consistent practice is key. Start with simple numerical examples, and then gradually move on to more complex real-life situations.

Simple Numerical Examples

Consider the following numerical examples:

7 > 2: In this comparison, seven is greater than two.

6 < 9: Here, six is less than nine.

Equation	Sign	Meaning	Description
7 > 2	>	Greater Than	Seven is greater than two
6 < 9	<	Less Than	Six is less than nine

As you practice more of these comparisons, you will get more comfortable with recognizing the signs and understanding their implications quickly.

Real-Life Examples

Let’s move on to more complex, real-life scenarios to understand these signs better:

• Suppose you have 20 apples and you give 15 to your friends. In this case, you’re left with fewer apples than you started with, represented as 20 > 15.

• Consider a car that has a speed limit of 60 mph but is currently traveling at 45 mph. The car is going at a speed less than the limit, represented as 45 < 60.

By practicing with these real-world examples, you can further improve your grasp of these signs and the relationships they represent.

Greater Than vs. Less Than: Practice Makes Perfect!

To sum it up, understanding the greater than and less than signs, and being able to use them correctly, is all about finding the method that resonates with you—be it the alligator method, the L method, or real-world scenarios—and practicing it regularly. With consistent effort, you will soon find that differentiating between the greater than and less than signs has become second nature to you.

We understand that math can be a challenging subject, and concepts like these often need more than just understanding—they require regular practice. Therefore, we encourage you to use our free math basics practice tests, study guides, and flashcards to further deepen your understanding of the greater than and less than signs, as well as other mathematical concepts that might be causing you trouble.

Our practice tests are designed to replicate the actual exam environment, helping you get accustomed to the types of questions you might encounter. Our study guides break down complex topics into easy-to-understand sections, and our flashcards provide a quick and efficient way to review and reinforce what you’ve learned.

Remember, every mathematical concept you master takes you one step closer to becoming proficient in the subject. And every bit of practice counts! So keep practicing, keep learning, and soon, you’ll find that these mathematical symbols and concepts are not just parts of a textbook, but tools you can use to understand and interpret the world around you.