Mathematics Study Guide for the ACT
Page 1
General Information
The ACT® test is designed to measure the level of learning for students at the end of the 11th grade. The test consists of 60 multiple-choice questions and must be completed within 60 minutes. Though this may seem a short time, this gives test-takers a minute per question, which should be more than enough time.
To maximize your efficiency, look over the test once, complete the questions that are easy to understand and solve, and then move on to the more difficult ones. This will allow you to deal with the easy items first, leaving more time for the questions you may struggle with later.
On the ACT test score report, you will receive one overall Math score and eight subscores. These scores will be derived from your performance on different questions that involve three areas of mathematics. The first area, shown below, is also divided into five categories of math (each of which you have had instruction on in high school or before), providing the five additional subscores.
These are the eight subscores you will receive in Math and the approximate percentage of questions from which each score will be derived. Note that a single question can contribute to more than one subscore.
Preparing for Higher Math (57% to 60%)
- Number and Quantity (7% to 10%)
- Algebra (12% to 15%)
- Functions (12% to 15%)
- Geometry (12% to 15%)
- Statistics and Probability (20% to 25%)
Integrating Essential Skills (40% to 43%)
These questions require you to apply skills you learned in math before the eighth grade to problems that also require higher level skills.
Modeling (More than 25%)
This score will be derived from your performance on any question that makes use of a model of some sort, whether you study a model, evaluate it, or devise how to produce it.
Within each of these subareas, these are the concepts with which you should be familiar. If there are any that give you trouble, seek extra practice on them before you take the ACT test.
Number and Quantity: Part 1
The Number and Quantity questions in the ACT Math section only comprise about 7% to 10% of the test, but these are the building blocks of the rest of the topics in math. In this section, we will look at the different types of numbers; doing calculations with different forms of numbers, factors, and multiples; rounding and place value; complex numbers; and many more topics.
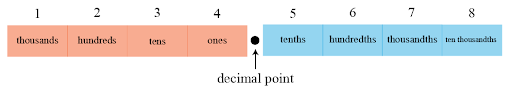
Place Value
Understanding place value is crucial for the concepts of Number and Quantity. Place value refers to the the value of a digit in a number based on its position within that number. Each digit in a multi-digit number has a place value based on its position and direction from the decimal point. The value of a digit increases as we move toward the left and decreases as we move toward the right. A place value chart is shown below:
Calculations
When performing calculations with different types of numbers, we follow certain rules. We are mainly interested in calculations with whole numbers, integers, and decimals. Rounding rules should also be noted.
Whole Numbers
Whole numbers are positive numbers that don’t have a fractional part (i.e., decimal part), as well as \(0\). We use whole numbers to count and measure. The whole numbers are \(0, \, 1, \, 2, \, 3, \, 4…\) We can easily perform addition, subtraction, multiplication, and division with whole numbers.
Integers
Integers are the whole numbers plus their negative counterparts (and \(0\)). The integers are \(...-3, \, -2, \, -1,\, 0, \, 1, \, 2, \, 3…\) They don’t include decimals or fractions. We use integers to represent quantities that are both positive and negative. As with whole numbers, we can perform the common mathematical operations on integers.
Decimals
Decimals are fractional numbers. They may have a whole part and a fractional part separated by a decimal point in the middle, or they may consist of only numbers to the right of the decimal point. Here are some examples of decimal numbers:
\(12.7\) (twelve and seven-tenths) \(100.41\) (one hundred and forty-one hundredths) \(0.451\) (four hundred fifty-one thousandths)
Ordering Decimals
If we are given a list of decimals, we can arrange them in ascending or descending order. To order decimal numbers, we compare the digits in each decimal starting from the left and moving to the right until we find a difference between the digits. The decimal number with the smaller digit at that point is the smaller of the two numbers.
Adding, Subtracting, Multiplying, and Dividing Decimals
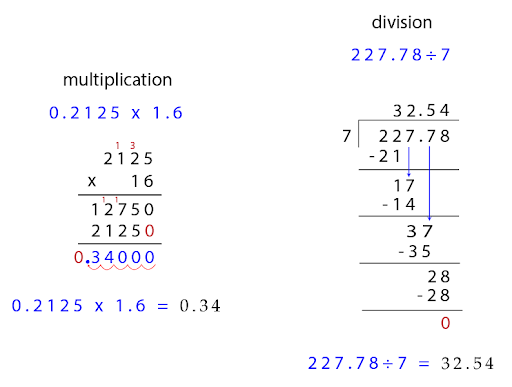
Adding, subtracting, multiplying, and dividing decimals are four fundamental operations that you need to master. To add or subtract decimals, you should line up the decimal points and then add or subtract as you would with whole numbers. Remember to keep the decimal point in the correct place in the answer. Also, use placeholder zeros when needed. Look at these two examples:

When multiplying decimals, multiply them as if they were whole numbers, and then count the number of decimal places in the problem and place the decimal point in the answer accordingly. When dividing decimals, you should move the decimal point in the divisor (the number you are dividing by) to the right until it becomes a whole number. Then, move the decimal point in the dividend (the number you are dividing) the same number of places to the right. Finally, perform the division as if the divisor and dividend were whole numbers and place the decimal point in the answer. Here are two examples:
Problems with Whole Numbers and Decimals
Problems involving whole numbers and decimals are quite common. To solve these types of problems, it is important to understand the relationships between these different types of numbers and how to perform basic operations with them. Here is one example:
Your restaurant bill comes to \(\$50.50\). If you leave a \(10\%\) tip, how much will you pay in total?
To solve this problem, first calculate the amount of the tip by multiplying the bill amount by \(0.10\) (\(10\%\) as a decimal), which gives you \(\$5.05\). Then, add the bill amount and the tip amount to get the total amount, which is \(\$55.55\).
Rounding Numbers
Rounding is the process of approximating a number to a certain degree of accuracy or precision. This is done by replacing the original number with a nearby number that is easier to work with or more appropriate for the situation. To round numbers, follow the following steps:
-
Determine the place value to which you want to round a number. We’ll call this the rounding digit.
-
Look at the digit to the right of the rounding digit. If the digit is \(5\) or greater, round the original number up by increasing the rounding digit by \(1\). If the digit to the right is less than \(5\), keep the rounding digit the same.
-
If the digit in the place value you are rounding to is \(9\) and you need to round up, carry the \(1\) over to the next digit to the left.
-
If you are rounding a decimal number to a whole number, simply drop all digits to the right of the decimal point.
All Study Guides for the ACT are now available as downloadable PDFs