
Formulas You Need to Know for the ACT Math Test
The ACT® (American College Testing) Math Test has 60 questions that cover a wide range of topics covered in high school. If you don’t remember your high school math classes, don’t worry! We’ve compiled the essential formulas you’ll need to know for the ACT Math Test. Even if you are not allowed to use this formula chart during the text, it’s a great tool for studying. Also, remember that in some cases, you’ll be allowed to use a calculator during your test, so you can remember the formulas and just plug in the values in your calculator.
Feel free to practice solving the problems on our sample ACT Math Test, and check out our study guide for the ACT Math section at Union Test Prep if you want more details.
Exponents
| Formula | Symbols |
|---|---|
| \(x^a \cdot x^b = x^{a+b}\) | \(a, b, x =\text{any real number}\) |
| \(\dfrac{x^a}{x^b}=x^{a-b}\) | \(a, b, x =\text{any real number}\) |
| \((x^a)^b=x^{a \cdot b}\) | \(a, b, x =\text{any real number}\) |
| \((x \cdot y)^a=x^a \cdot y^a\) | \(a, x, y =\text{any real number}\) |
| \(x^1=x\) | \(x= \text{any real number}\) |
| \(x^0 = 1\) | \(x = \text{any real number}\) |
| \(x^{-a} = \dfrac{1}{x^a}\) | \(a,x = \text{any real number}\) |
| \(x^{\frac{a}{b}} = \sqrt[b]{x^a} = (\sqrt[b]{x})^a\) | \(a, b, x = \text{any real number}\) |
Statistics
| Formula | Symbols |
|---|---|
| \(p = \dfrac{d}{t}\) | \(p = \text{probability of an event}\) \(d = \text{desired event}\) \(t= \text{total number of possible events}\) |
| \(\bar{x} = \dfrac{\sum{x_i}}{n}\) | \(\bar{x} = \text{mean}\) \(x_i = \text{value of each measurement}\) \(n = \text{number of measurements}\) |
| \(s = \sqrt{\mathstrut \dfrac{\sum(x_i - \bar{x})^2}{n-1}}\) | \(s = \text{standard deviation}\) \(\bar{x}=\text{mean}\) \(x_i = \text{value of each measurement}\) \(n = \text{number of measurements}\) |
| \(V = s^2\) | \(V = \text{variance}\) \(s = \text{standard deviation}\) |
| \(CV = RSD = 100 \cdot \dfrac{s}{\bar{x}}\) | \(CV = \text{coefficient of variation}\) \(RSD = \text{relative standard deviation}\) \(s = \text{standard deviation}\) \(\bar{x} = \text{mean}\) |
Linear Equations
| Formula | Symbols | Comments |
|---|---|---|
| \(A\cdot x + B\cdot y = C\) | \(A, B, C = \text{any real number}\) \(y= \text{dependent variable}\) \(x = \text{independent variable}\) |
Standard Form |
| \(y=m \cdot x + b\) | \(y = \text{dependent variable}\) \(m= \text{slope}\) \(x = \text{independent variable}\) \(b = y \text{-intercept}\) |
Slope-Intercept Form. Try to convert linear equations to this format. |
| \(m = \dfrac{y_2 - y_1}{x_2 - x_1}\) | \(m = \text{slope}\) \(y_n = \text{dependent variable (point n)}\) \(x_n = \text{independent variable (point n)}\) |
This is a rearranged version of the point-slope form. |
| \(y-y_1 = m(x-x_1)\) | \(y= \text{dependent variable}\) \(x = \text{independent variable}\) \(y_1 = y \text{ value of a point on the line}\) \(x_1 = x \text{ value of a point on the line}\) \(m = \text{slope}\) |
Point-Slope form |
| \(x+a = b \Rightarrow x = b-a\) \(x-a = b \Rightarrow x = b+a\) \(x \cdot a = b \Rightarrow x = b \div a\) \(x \div a = b \Rightarrow x = b \cdot a\) \(x^a = b \Rightarrow x = \sqrt[a]{b}\) \(\sqrt[a]{x} = b \Rightarrow x = b^a\) \(a^x = b \Rightarrow x = \dfrac{\log b}{\log a}\) |
\(a, b = \text{constants}\) \(x = \text{variable}\) |
Quadratic Equations
| Formula | Symbols | Comments |
|---|---|---|
| \(x= \frac{-b \pm \sqrt{b^2-4ac}}{2a}\) | \(a,b = \text{constants}\) \(c = \text{constant (y-intercept)}\) \(x = \text{variable}\) |
Quadratic Formula for equation in the form \(ax^2+bx+c=0\) |
| \((a \pm b)^2 = a^2 \pm 2ab + b^2\) | \(a,b = \text{constants or variables}\) | Square of a sum or difference |
| \(a^2-b^2 = (a+b)\cdot (a-b)\) | \(a,b = \text{constants or variables}\) | Difference of squares |
Cubic Equations
| Formula | Symbols | Comments |
|---|---|---|
| \(a^3-b^3 = (a-b) \cdot (a^2+ab + b^2)\) | \(a,b = \text{constants or variables}\) | Difference of cubes |
| \(a^3+b^3 = (a+b) \cdot (a^2-ab + b^2)\) | \(a,b = \text{constants or variables}\) | Sum of cubes |
Sequences and Patterns
| Formula | Symbols | Comment |
|---|---|---|
| \(a_n=a_1+(n-1) \cdot d\) | an = value of the nth term a1 = first term n = any number in the series d = difference b/w consecutive terms |
This formula is for an arithmetic sequence |
| \(s_n=\frac{n \cdot (a_1 + a_n)}{2}\) | sn = sum of sequence with n terms an = value of the nth term a1 = first term n = any number in the series |
This formula is for an arithmetic sequence |
| \(r = \frac{a_2}{a_1} = \frac{a_n}{a_{(n-1)}}\) | r = ratio in a geometric sequence a1 = first term a2 = second term an = value of the nth term |
This formula is for a geometric sequence |
| \(a_n=a_1 \cdot r^{n-1}\) | r = ratio in a geometric sequence a1 = first term n = any number in the series an = value of the nth term |
This formula is for a geometric sequence |
| \(s_n = \frac{a_1 \cdot (1-r^n)}{1-r}\) | r = ratio in a geometric sequence a1 = first term n = any number in the series sn = sum of sequence with n terms |
This formula is for a geometric sequence |
Functions
| Formula | Symbols | Comment |
|---|---|---|
| \((f+g)(x) = f(x) + g(x)\) | \(f(x) = \text{function } f\) \(g(x) = \text{function } g\) |
Addition of Functions |
| \((f-g)(x) = f(x) - g(x)\) | \(f(x) = \text{function } f\) \(g(x) = \text{function } g\) |
Subtraction of Functions |
| \((f \cdot g)(x) = f(x) \cdot g(x)\) | \(f(x) = \text{function } f\) \(g(x) = \text{function } g\) |
Multiplication of Functions |
| \((\frac{f}{g})(x) = \frac{f(x)}{g(x)}\) | \(f(x) = \text{function } f\) \(g(x) = \text{function } g\) |
Division of Functions |
| \((f \circ g)(x) = f(g(x))\) | \(f(x) = \text{function } f\) \(g(x) = \text{function } g\) |
Composition of Functions |
Geometry Equations
| Formula | Symbols | Comments |
|---|---|---|
| \(A = s^2\) | \(A = \text{area of a square}\) \(s = \text{side length}\) |
|
| \(A = l \cdot w\) | \(A = \text{area of a rectangle}\) \(l = \text{length}\) \(w = \text{width}\) |
|
| \(A = \dfrac{1}{2} b \cdot h\) | \(A = \text{area of a triangle}\) \(b= \text{base}\) \(h = \text{height}\) |
|
| \(A = \pi \cdot r^2\) | \(A = \text{area of a circle}\) \(r = \text{radius}\) |
|
| \(A = h \cdot \dfrac{b_1+b_2}{2}\) | \(A = \text{area of a trapezoid}\) \(b_n = \text{base }n\) \(h = \text{height}\) |
|
| \(C= 2 \pi r = \pi d\) | \(C = \text{perimeter of a circle}\) \(r = \text{radius}\) \(d = \text{diameter}\) |
|
| \(V = s^3\) | \(V = \text{volume of a cube}\) \(s = \text{side length}\) |
|
| \(V = l \cdot w \cdot h\) | \(V = \text{volume of a rectangular prism}\) \(l = \text{length}\) \(w = \text{width}\) \(h = \text{height}\) |
|
| \(V = \dfrac{4}{3} \pi r^3\) | \(V = \text{volume of a sphere}\) \(r = \text{radius}\) |
|
| \(V = \pi r^2 h\) | \(V = \text{volume of a cylinder}\) \(r = \text{radius of base}\) \(h = \text{height}\) |
|
| \(V = \dfrac{1}{3} \pi r^2 h\) | \(V = \text{volume of a cone}\) \(r = \text{radius}\) \(h = \text{height}\) |
|
| \(V = \dfrac{1}{3} l \cdot w \cdot h\) | \(V = \text{volume of a pyramid}\) \(l = \text{length}\) \(w = \text{width}\) \(h = \text{height}\) |
|
| \(d= \sqrt{\mathstrut (y_2 - y_1)^2 + (x_2-x_1)^2}\) | \(d = \text{distance between two points}\) \(y_n = y \text{ value at point n}\) \(x_n = x \text{ value at point n}\) |
|
| \(a^2 + b^ 2 = c^ 2\) | \(a,b = \text{legs of a right triangle}\) \(c = \text{hypotenuse of a right triangle}\) |
Pythagorean theorem |
| \((x-h)^2 + (y-k)^2 = r^2\) | \((h,k) = \text{center of a circle}\) \(r = \text{radius}\) |
Standard form of a circle |
| \(x^2 + y^2 + Ax + By + C = 0\) | \(x, y = \text{variables}\) \(A,B,C = \text{constants}\) |
General form of a circle |
Trigonometry Equations
| Formula | Symbols | Comments |
|---|---|---|
| \(\sin^2 \theta + \cos^2 \theta = 1\) | Pythagorean Identity | |
| \(\sin 2\theta = 2 \sin \theta \cdot \cos \theta\) \(\cos 2\theta = \cos^2 \theta - \sin^2 \theta = 2 \cos^2 \theta -1\) \(\tan 2\theta = \frac{2 \tan \theta}{1-\tan^2 \theta}\) |
Double Angle formulas |
Formulas with Diagrams
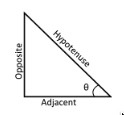
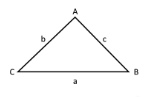
Keep Reading

ACT Blog
Essay Writing Practice and Prompts for the ACT
The ACT writing test is an optional exam, and is not always given as pa…

ACT Blog
How to Do Well on the ACT Essay
Understanding the ACT Essay Before diving into strategies to excel, it…

ACT Blog
How to Study for the ACT in One Week
Getting ready for American College Testing (the ACT) can be a source of…