Math Study Guide for the SAT Exam

Additional Statistical Concepts

Confidence Interval

A confidence interval describes both the degree of accuracy and the uncertainty of an estimated value. On the SAT exam, confidence intervals will be given, and it’s a usually 95% confidence level.

What does it mean when the sample mean height is 121 cm and the margin of error is 1.3 cm at 95% confidence level?

This statement can be interpreted as:

There is 95% confidence that the true average height for the entire population is within the interval 119.7 cm to 122.3 cm. If the same method of estimating the parameter and size of the random sample were repeatedly performed, the actual average height will be within 119.7 cm to 122.3 cm 95% of the time.

Important note:

The confidence interval applies to the parameter (e.g., the mean height of the entire population) and not to the value of the other variable (e.g., the number of individuals). In other words, the illustration above cannot be interpreted as: 95% of the population have a height between 119.7 cm and 122.3 cm.

Univariate vs. Bivariate Data

Univariate data refers to data sets with one type of variable, such as the number of hot beverages sold by a café. The variable is the number of each type of hot beverage sold. It can be shown in this data set:

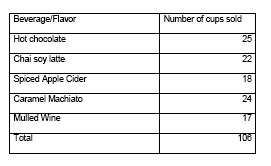
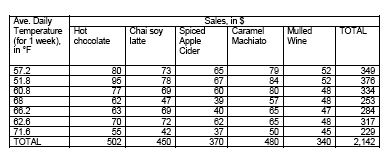
Bivariate data refers to data sets with two types of variables. If the café owner wanted to find a relationship between their sales on a particular day and the temperature on that day, bivariate data can be gathered, such as their sales of the five hot beverages versus the temperature for each day of the week. The variables are the sales and the temperature. It will look something like this:

Linear, Quadratic, and Exponential Relationships

Variables have a linear relationship when they increase or decrease at a constant rate. As one variable increases the other one decreases, and vice versa. The difference between two adjacent values is constant. When plotted, this is represented by a straight line sloping up or down.

A U-shaped graph facing either upward or downward indicates a quadratic relationship. The rate of change is variable. There’s either a maximum or minimum value which is seen in the graph as the vertex.

A graph that starts to change very gradually initially (either increasing or decreasing), but suddenly takes a significant change over time, indicates an exponential relationship. An exponential curve does not have a vertex.

Variability

Parameters and statistics are estimates used to describe a population or a sample of a population. The numerical values, though, are not the exact actual values but are only the closest estimate. The variability of an estimate against actual values must be accounted for, and this is done by calculating measures of spread.

The spread or scatter of data in a set is measured in various ways, and the most common measures are: range, interquartile range (IQR), variance, and standard deviation. These are ways of describing spread in relation to the estimated value.

Randomization

A random sample truly represents its population if it was selected by a purely chance method, also called randomization, and every element of the population has not been excluded in the procedure. By this, we mean that every element of the population has a probability of being included in the sample, and the whole process is protected from biases.

Some of the methods are: using random numbers (e.g., random number table or random number generator, flipping a coin, or throwing a die).

These are important things to remember:

Random sampling is necessary so that the result of an experiment can be generalized to the entire population.
Random assignment of the subjects to different treatments is also necessary to ensure that all subjects started under generally the same condition before they were subjected to any treatment. This makes it appropriate to draw conclusions about the cause and effect of each treatment.

In the SAT exam, a question may describe a situation regarding the manner of selecting subjects and the manner of assigning them to treatments. The question may then ask which statements can be appropriately drawn from the experiment.

Specific Problem-Solving Skills to Practice

In addition to knowing what math terms mean, you’ll need to be able to use them appropriately and accurately to solve problems. If any of these procedures are difficult for you, seek additional practice until you become fluent and accurate in their use.

Solve a Multistep Problem

Just knowing a single procedure is not enough to answer most questions on this test. The SAT exam is really a test of reasoning, as much as math, and a problem often involves several procedures along the way. Here are some examples.

Use a Proportion to Determine a Ratio and Rate

Ratios that are equal are said to be proportionate to each other, such as $\frac{5}{7}$ and $\frac{25}{35}$.

A bowl contains a mixture of $1$ cup cornstarch and $4$ cups flour. A second bowl contains the same ingredients in the same proportion, but instead has $1.5$ cups of cornstarch. If $\frac{1}{2}$ cup of oats is added to the second bowl, what is the ratio of oats to flour in the second bowl?

First, determine the amount of flour in the second bowl, using the same ratio in the first bowl (which is $1:4$). We represent the amount of flour with the variable $x$. Ratios are in proportion if they are equal, so:

\[\frac{1}{4} = \frac{1.5}{x}\] \[x = 6\]

The ratio of oats to flour, then is $0.5$ is to $6$, or $1:12$.

#### Use Ratio and Rate to Solve a Multistep Problem

Questions involving ratio and proportion usually ask for an unknown part or component. See this example:

The concrete mix to a floor slab must follow the ratio of $1$ part cement, to $3$ parts sand, to $3$ parts stone aggregates. How many buckets of cement ($C$) will be needed for a total of $10$ buckets of sand and $10$ buckets of stone aggregates?

The ratio is $1:3:3$, and we need to find $C$ for $C:10:10$.

We may just use the first two parts of each ratio and set the two ratios equal to each other:

\[\frac{1}{3} = \frac{C}{10}\] \[\frac{10}{3} = C\] \[C = 3 \frac{1}{3}\]

Calculate Percentage, then Solve a Problem

Andy usually buys large Grade A eggs from her supplier at $\$2.50$ per dozen. She chanced upon a farm where the eggs were sold at $70\%$ of this price and bought $10$ dozens. How much more would she have paid for the $10$ dozens of eggs had she bought from her regular supplier?

First, calculate the price of eggs at the farm, which is $70\%$ of $\$2.50$:

Price at the farm = $0.7 \times 2.50 = \$1.75$

Then, we proceed to answer the question being asked. We need to know the price she paid for the whole purchase, the price she would have paid had she bought from her regular supplier, and then solve for the amount she saved.

$\$1.75$ is the price for one dozen. She bought ten dozens, so the amount she paid was:

Total amount paid = $1.75 \times 10 = $17.50$

The price for ten dozen eggs from her regular supplier:

Price from regular supplier = $2.50 \times 10 = \$25.00$

Getting the difference of the two total amounts, we get Andy’s savings:

Amount Andy saved = $25 – 17.50 = \$7.50$

Use Unit Conversion

Familiarity with the unit conversion method, also called the factor-label method, comes in very handy when dealing with many rate questions and when double-checking answers. Let’s use this method in an actual rate question.

Alia has an annual basic salary rate of $\$53,760$. If she works $8$ hours a day, four days in a week, and four weeks in a month, what is her hourly rate?

Even without a formula, this can be solved using the unit conversion method.

Start with the given information and take note of the final unit of measure being asked in the question:

$\$53,760$/year = __/hour

Using the unit conversion method, simply multiply the given with the conversion factors until you get to the required unit of measurement:

\[\frac{\$53760}{\text{year}} \cdot \frac{1\text{year}}{12 \text{months}} \cdot \frac {1\;\text{month}}{4\;\text{weeks}} \cdot \frac{1\;\text{week}}{4\;\text{days}} \cdot \frac{1\;\text{day}}{8\;\text{hours}} = \frac{\$35}{\text{hour}}\]

Take note that we deliberately wrote conversion factors in such a manner that similar units canceled out, for example, week units were written as numerator and denominator so that they canceled each other out. This is to say that you may write conversion factors in any way that makes it favorable for you to cancel units out.

The same is true with the other units we wanted to disappear, leaving us only with the units required in the answer. Also, the conversion factors used were those specifically given in the question, and not those we typically know, such as $1$ day = $24$ hours.

Match Graphs to Properties and Values

You need to sharpen your skills on matching graphs to the properties and values of a data set.

Categorical data, such as the genre of music preferred by students in a high school, are appropriately presented by pie and bar graphs.

Numerical data, which are either discrete or continuous, are plotted using line graphs, histograms, and scatterplots, such as Company A’s human resource department’s annual expenditures over a 10-year period.

On the SAT exam, you may be given a graph and asked to interpret it. You will need to understand what you see graphically and relate this to important features—the center, spread, and shape.

Use Data to Make Inferences

Inferences about the population can be made from the results of sample surveys as long as random sampling has been used for the study.

Take this statement for example:

In a survey based on a random sample of students in XY Senior High School, 68% said that they spend at least $7$ hours a day on social networks.

You can correctly infer that “About $68\%$ of all students in XY Senior High School spend at least $7$ hours a day on social networks.”

Here’s another inference that can be made:

If the random sample consisted of $100$ students out of $1,250$ students and $12$ of those surveyed said that they spent less than $3$ hours a day on social networks, about how many students at XY Senior High spend less than $3$ hours a day on social networks?

A reasonable estimate would be $150$ students:

\[\frac {12}{100} = \frac {N}{1250}\] \[N = 150\]

Draw Conclusions from Data

A well-designed survey makes it possible to cut the cost and time necessary for a census and still obtain a result that can be generalized to the entire population. Through random sampling, all elements of the population have a probability of being selected, and the result is protected from biases.

Both experiments and observational studies investigate the causal relationship between a dependent variable and an independent variable. In experiments, the researcher has control over the participant’s assignment to groups and treatments given to each group; hence, random assignment of subjects can be done. This control is absent in observational studies, and findings derived from this method cannot be used for causal inference and generalization to the larger population.

Justify Conclusions with Data

Questions in the SAT could ask for the validity of a conclusion based on the data gathered.

Situations could be given such as:

A study proposes the use of a module (let’s say Module A) in a senior high school to improve students’ competency in the area of mathematics. Two other modules were included in the study, including the current module being officially used by the school (Module B). Three groups, each made up of 2 classrooms of Year 11 students, were assigned a module. After two months, students were selected from each group to take a test designed to measure their improvement. A table summarizing the test results could be given in the question, along with other information, from which you can justify the conclusion drawn.

Be prepared to interpret data given in tables and graphs. Do the given data support the conclusion? How were students who took the test chosen? Were there other variables that could have contributed to the test result but were not included in the study? Because the given example refers to a study of cause and effect, were the proper controls in place (i.e., random assignment of subjects)?

These questions must be asked first by the person or the group conducting the study to make sure that their data justify their conclusions.

Evaluate Data Collection Methods

It is important to know how the data in a study was obtained because this largely determines whether it is appropriate to draw and apply conclusions to the entire population.

Data are obtained by conducting a census (entire population), survey (random sample), experimental study (cause-and-effect, controlled), and observational study (cause-and-effect, not controlled).