Formulas for the Math Section of the CBEST® Test

There’s ONE single skill you have to master if you want to ace the CBEST® Math test: Reading.

Are you thinking that we are mistaken? Think again. Reading is crucial for solving all the problems you’ll face during the Math Section of the CBEST® Test, because all the questions will be given to you in words, not numbers. Reading skills are also important when studying for the test, since you need to understand the fundamental concepts of math if you want to succeed.

That’s why we have plenty of reading material for you and it’s all FREE. You can read 6 pages of preparatory material here:

Union Test Prep’s Study Guide for the CBEST® Math Section

Or you can browse through these to see how ready you are:

Practice Questions

Flashcards

Or, if you are in the mood for light reading, you can check the following formula chart, which has the essential content you need to know before the test. Also use these when accessing our practice questions and flashcards for this test.

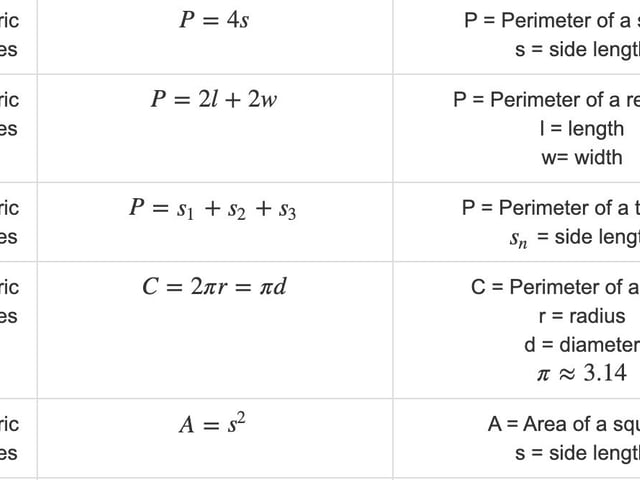
Math Formulas for the CBEST

Category	Formula	Symbols	Comment
Geometric Quantities	\(P = 4s\)	P = Perimeter of a square s = side length
Geometric Quantities	\(P = 2l + 2w\)	P = Perimeter of a rectangle l = length w= width
Geometric Quantities	\(P = s_1 + s_2 + s_3\)	P = Perimeter of a triangle \(s_n\) = side length
Geometric Quantities	\(C = 2 \pi r = \pi d\)	C = Perimeter of a circle r = radius d = diameter \(\pi \approx 3.14\)
Geometric Quantities	\(A = s^2\)	A = Area of a square s = side length
Geometric Quantities	\(A = lw\)	A = Area of a rectangle l = length w = width
Geometric Quantities	\(A = bh\)	A = Area of a parallelogram b = base h = height
Geometric Quantities	\(A = \frac{1}{2} bh\)	A = Area of a triangle b = base h = height
Geometric Quantities	\(A = h \cdot \dfrac{b_1 + b_2}{2}\)	A = Area of a trapezoid \(b_n\) = base n h = height
Geometric Quantities	\(A = \pi r^2\)	A = Area of a circle r = radius \(\pi \approx 3.14\)
Volume	\(V=lwh\)	V = Volume of a rectangular prism l = length w = width h = height
Volume	\(V= Bh\)	V = Volume of a right prism B = area of the base h = height
Volume	\(V = \pi r^2 h\)	V = Volume of a cylinder r = radius h = height \(\pi \approx 3.14\)
Volume	\(V = \frac{1}{3} B h\)	V = Volume of a pyramid B = area of the base h = height
Volume	\(V = \frac{1}{3} \pi r^2 h\)	V = Volume of a cone r = radius h = height \(\pi \approx 3.14\)
Volume	\(V = \frac{4}{3} \pi r^3\)	V = Volume of a sphere r = radius \(\pi \approx 3.14\)
Statistics	\(p = \frac{d}{t}\)	p = probability of an event d = desired event t = total number of possible events
Statistics	\(\overline{x} = \dfrac{\Sigma x_i}{n}\)	\(\overline{x}\) = mean \(x_i\) = value of each measurement n = number of measurement
Statistics	\(s = \sqrt{ \dfrac{ \Sigma (x_i - \overline{x})^2}{n-1}}\)	s = standard deviation \(\overline{x}\) = mean \(x_i\) = value of each measurement n = number of measurements
Statistics	\(V=s^2\)	V = Variance s = standard deviation
Statistics	\(CV = RSD = 100 \cdot \frac{s}{\overline{x}}\)	CV = Coefficient of variation RSD = Relative standard deviation s = standard deviation \(\overline{x}\) = mean
Fractions	\(\frac{a}{b} + \frac{c}{d} = \frac{ad + cb}{bd}\)	a,b,c,d = any real number	Remember to Simplify the fraction (if possible)
Fractions	\(\frac{a}{b} \cdot \frac{c}{d} = \frac{ac}{bd}\)	a,b,c,d = any real number	Remember to Simplify the fraction (if possible)
Fractions	\(\frac{a}{b} \div \frac{c}{d} = \frac{ad}{bc}\)	a,b,c,d = any real number	Remember to Simplify the fraction (if possible)
Fractions	\(a \frac{b}{c} = \frac{ac + b}{c}\)	a,b,c = any real number	Remember to Simplify the fraction (if possible)
Percents	\(a \cdot b \% = a \cdot \frac{b}{100}\)	a = any real number b% = any percent	Remember to simplify (if possible)
Percents	\(\% = \dfrac{\lvert b-a \rvert }{b} \cdot 100 = \frac{c}{b} \cdot 100\)	% = % increase or decrease a = new value b = original value c = amount of change