$Formula List for the PERT Mathematics Test$

Formula List for the PERT Mathematics Test

Taking the PERT Mathematics Test? One key to success is knowing your formulas. Think of them as shortcuts to solving problems. Yes, understanding the bigger concepts is important, but during a timed test, quickly recalling a formula can save you valuable time. In the sections below, we’ll explore why these formulas are so crucial, provide a handy list of the ones you’ll likely need, and give you tips on how to remember them. Let’s get started and set you up for success!

Why Memorizing Formulas is Crucial for the PERT Mathematics Test

Builds a Strong Foundation

Remember that math is hierarchical in nature. Basic concepts serve as a foundation for more complex problems. By memorizing these essential formulas, you are essentially cementing your foundational understanding of the subject. This makes it easier to solve both straightforward and complex problems on the test.

Enhances Efficiency

While the PERT Mathematics Test doesn’t have a strict time limit, being efficient is still crucial. Knowing formulas off the top of your head means you don’t have to spend extra time deriving or recalling them. This lets you focus more on understanding and solving the problem, ensuring a smoother testing experience.

Reduces Test Anxiety

Knowing that you have prepared thoroughly and have a handy list of formulas can greatly reduce test-related anxiety. Confidence in your knowledge and abilities can enhance your performance on the day of the test.

Essential Formulas for the PERT Mathematics Test

To underscore the importance of formulas in your preparation for the PERT Mathematics Test, here’s a curated list of essential formulas. This chart acts as a quick reference point, enabling you to familiarize yourself with critical mathematical tools you’ll encounter during the exam. Keep it handy as you navigate through the subsequent sections.

Formulas for the PERT Mathematics Test

Category	Formula	Symbols	Comment
Arithmetic	$a+b=b+a$ $a \cdot b = b \cdot a$	a, b = any constant or variable	Commutative Property
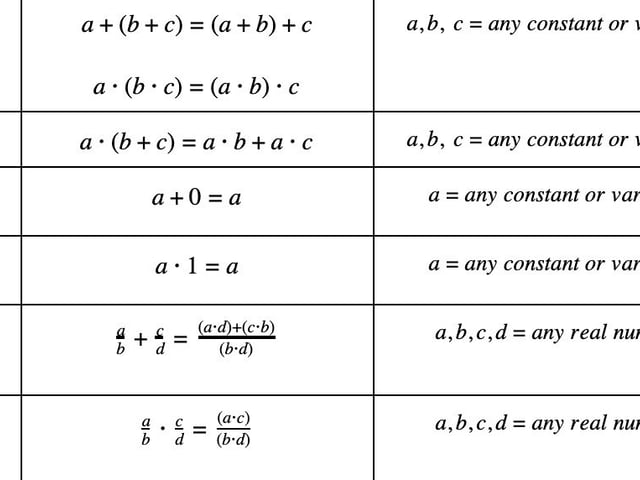
Arithmetic	$a+(b+c)=(a+b)+c$ $a \cdot (b \cdot c)=(a \cdot b) \cdot c$	a, b, c = any constant or variable	Associative Property
Arithmetic	$a \cdot (b+c)=a \cdot b + a \cdot c$	a, b, c = any constant or variable	Distributive Property
Arithmetic	$a+0=a$	a = any constant or variable	Identity Property of Addition
Arithmetic	$a \cdot 1 = a$	a = any constant or variable	Identity Property of Multiplication
Arithmetic	$\dfrac{a}{b} + \dfrac{c}{d} = \dfrac{(a \cdot d)+(c \cdot b)}{(b \cdot d)}$	a, b, c, d = any real number	Remember to simplify the fraction if possible.
Arithmetic	$\dfrac{a}{b} \cdot \dfrac{c}{d}=\dfrac{a \cdot c)}{(b \cdot d)}$	a, b, c, d = any real number	Remember to simplify the fraction if possible.
Arithmetic	$\dfrac{a}{b} \div \dfrac{c}{d}=\dfrac{a \cdot d)}{(b \cdot c)}$	a, b, c, d = any real number	Remember to simplify the fraction if possible.
Arithmetic	$a\dfrac{b}{c}=\dfrac{(a \cdot c)+b}{c}$	a, b, c = any real number	Remember to simplify the fraction if possible.
Algebra	$x+a=b \Rightarrow x=b-a$ $x-a=b \Rightarrow x=b+a$ $x \cdot a=b \Rightarrow x=b \div a$ $x \div a=b \Rightarrow x=b \cdot a$ $x^a=b \Rightarrow x = \sqrt[a]{b}$ $\sqrt[a]{x}= b \Rightarrow x= b^a$ $a^x=b \Rightarrow x=\frac{log\ b}{log\ a}$	a, b = constants x = variable
Algebra	$x^a \cdot x^b=x^{a+b}$	a, b, x = any real number
Algebra	$\dfrac{x^a}{x^b}=x^{a-b}$	a, b, x = any real number
Algebra	$(x^a)^b =a^{a \cdot b}$	a, b, x = any real number
Algebra	$(x \cdot y)^a = x^a \cdot y^a$	a, b, y = any real number
Algebra	$x^1=x$	x = any real number
Algebra	$x^0=1$	x = any real number
Algebra	$x^{-a} = \dfrac {1}{x^a}$	a, x = any real number
Algebra	$x^{\frac {a}{b}} = \sqrt[b]{x^a} = (\sqrt[b]{x})^a$	a, b, x = any real number
Algebra	$\dfrac{x}{\sqrt{y}} \cdot \dfrac {\sqrt{y}}{\sqrt{y}} = \dfrac{x \sqrt{y}}{y}$	x, y = any real number
Linear Equations	$A \cdot x + B \cdot y=C$	A, B, C = any real number y = dependent variable x = independent variable	Standard Form
Linear Equations	$y=m \cdot x + b$	y = dependent variable m = slope x = independent variable b = y-axis intercept	Slope-intercept form Try to convert any linear equation to this form.
Linear Equations	$m = \dfrac{(y_2 - y_1)}{(x_2 - x_1)}$	m = slope $y_n$ = independent variable (point n) $x_n$ = dependent variable (point n)	This is a rearrangement of the point-slope form.
Linear Equations	$y-y_1 = m(x-x_1)$	$(x_1,y_1)$ = point on the line y = dependent variable x = independent variable m = slope y = independent variable x = dependent variable	Point-Slope Form
Quadratic Equations	$x= \dfrac{-b \pm \sqrt{b^2-4 \cdot a \cdot c}}{2 \cdot a}$	a, b, c = constants c = y-axis intercept x = variable (x intercepts)	Quadratic Formula for equation in the form $ax^2+bx+c=0$
Quadratic Equations	$(a \pm b)^2 = (a^2 \pm 2 \cdot a \cdot b+b^2)$	a, b = constants or variables	Square of sum or difference
Quadratic Equations	$a^2 - b^2 = (a+b)(a-b)$	a, b = constants or variables	Difference of squares
Percents	$a \cdot b\%=a \cdot \dfrac{b}{100}$	a = any real number b% = any percent	Remember to simplify if possible
Percents	$\% = \dfrac{\vert b-a \vert}{b} \cdot 100= \dfrac{c}{b} \cdot 100$	% = % increase or decrease a = new value b = original value c = amount of change

Tips for Memorizing and Utilizing Formulas Effectively

Use Mnemonics and Acronyms

A mnemonic is a memory aid. By creating catchy rhymes, stories, or acronyms for complex formulas, you can make them easier to recall during the test. For example, “PEMDAS” (Please Excuse My Dear Aunt Sally) is a well-known acronym to remember the order of operations in mathematics: Parentheses, Exponents, Multiplication, Division, Addition, and Subtraction.

Practice Regularly

Simply memorizing the formulas isn’t enough. Make sure you apply them regularly in practice problems. The more you use them, the more ingrained they’ll become in your memory.

Teach Someone Else

They say the best way to learn is to teach. Explain these formulas to a friend or a study buddy. This not only reinforces your own understanding but also highlights areas you might need to work on. For instance, if you’re teaching the Pythagorean theorem and find it challenging to explain, it might be a sign that you need to revisit and solidify your own comprehension.

Flashcards

Utilize flashcards to test your formula recall. On one side, write a brief description or a related problem, and on the other, the formula. Regularly test yourself with these to improve retention.

Beyond Formulas: Delving Deeper into Key Concepts

Formulas in mathematics are akin to tools in a toolbox. While they are crucial for problem-solving, understanding the underlying principles behind each formula is what ensures effective application, especially in a dynamic test environment. Here, we’ll explain some of these core principles and concepts for a few select formulas:

Algebra

Exponential Laws

1) Formula: $x^a \cdot x^b = x^{a+b}$

This formula represents the product of powers rule in exponents. When you multiply two exponential terms with the same base, you simply add their exponents.

Example problem: simplify the expression: $3^4 \cdot 3^2$

Solution: $3^4 \cdot 3^2 = 3^{4+2} = 3^6$

2) Formula: $\frac{x^a}{x^b} = x^{a-b}$

This is the quotient of powers rule. When dividing two exponential terms with the same base, you subtract the exponents.

Example problem: simplify the expression: $\frac{5^7}{5^3}$

Solution: $\frac{5^7}{5^3} = 5^{7-3} = 5^4$

3) Formula: $(x^a)^b = x^{a \cdot b}$

This is the power of a power rule. When raising an exponential term to another power, you multiply the exponents together.

Example problem: simplify the expression: $(2^3)^4$

Solution: $(2^3)^4 = 2^{3 \cdot 4} = 2^{12}$

Arithmetic

Properties of Numbers

Formula: $\frac{a}{b} + \frac{c}{d} = \frac{a \cdot d + c \cdot b}{b \cdot d}$

This formula represents the process of adding fractions with different denominators. To add them, you need a common denominator. The quickest way to get one is to multiply the two denominators together.

Example Problem: Add the following fractions: $\frac{2}{3} + \frac{4}{5}$

Solution: $\frac{2}{3} + \frac{4}{5} = \frac{2 \cdot 5 + 4 \cdot 3}{3 \cdot 5} = \frac{10 + 12}{15} = \frac{22}{15}$

Percents

Formula: $a \cdot b\% = a \cdot \frac{b}{100}$

This formula transforms a percentage into a decimal. Percent means “out of 100.” So, multiplying a number by a percentage is the same as multiplying it by the decimal form of that percentage.

Example Problem: Find 40% of 150:

\[150 \cdot 40\%\]

Solution: $150 \cdot 40\% = 150 \cdot \frac{40}{100} = 60$

Real-world Applications

Every mathematical concept has roots in real-life scenarios:

Exponential Laws: Think about compound interest in banking. The way your savings grow over time, especially when interest is compounded annually, monthly, or even daily, is governed by the rules of exponents.
Arithmetic with Fractions: Imagine you’re cooking and need to add differently measured ingredients. Combining $\frac{1}{3} \text{ cup}$ and $\frac{1}{4} \text{ cup}$ requires the understanding of adding fractions with different denominators.
Percents: Whether it’s calculating discounts during a sale, understanding tax rates, or even gauging the growth of your investments, percentages play a pivotal role in financial literacy.

Understanding these foundational concepts ensures not only success in the PERT Mathematics test but also better numerical literacy in everyday life.

Final Thoughts

The PERT Mathematics test can be a challenging hurdle, but with the right preparation, you can certainly ace it. By memorizing the essential formulas and understanding the underlying concepts, you’re setting yourself up for success. Remember to use all the resources available to you, including the practice questions, flashcards, and study guides provided by Union Test Prep. Happy studying and best of luck!