Test II Mathematics Study Guide for the GACE

General Information

About 53% of the GACE Elementary Education Test II (002) is made up of math questions. Most of the questions are typical multiple-choice questions, but you may also see a few fill-in-the-blank and/or multiple-answer multiple-choice questions.

Yes, it’s vital to know the content in this study guide, but equally important to be able to match appropriate teaching strategies to each concept and skill. It would be a really good idea to extract these strategies from either your memory or some other resources, making a note of them while you go through the concepts in this study guide.

Counting and Cardinality

The rote memorization of number words in order is a key foundational mathematics skill that allows students to count and understand cardinality. Students understand and apply knowledge of these words to say a number name, in order, for each object in a set to tell how many. Often, strong counting skills precede the understanding of cardinality.

Cardinality is the knowledge that the last number said when counting notes the number of objects in the set. As student counting skills become more sophisticated, they may use skip counting (counting by 2s, 5s, or any predetermined pattern), counting on (starting to count at a number other than 0), or counting backward.

A strong understanding of counting and cardinality sets students up for success in all areas of mathematics.

Counting

Students answer the question “how many” by saying the names of numbers in the correct order to mark items in a set. To accurately mark, they must use one-to-one correspondence.

One-to-one correspondence is the knowledge that one number name represents one (and only one) object in the set. If a student skips an object, counts an object twice, or says a number name without touching or counting an object, they do not yet have one-to-one correspondence.

Strategies to build one-to-one correspondence include touching objects while counting, putting up/taking down fingers, and clapping or stepping when saying a number.

Matching Numbers to Quantities

A strong understanding of one-to-one correspondence and cardinality sets students up to match numbers to quantities. Students can match written or oral numbers to sets by counting or subitizing.

Subitizing is the process of recognizing the quantity of a set instantly, without counting each object individually. Students should also understand the concept of number conservation, the fact that a quantity of a group remains the same regardless of changes to its physical arrangement.

Comparing Numbers and Quantities

Students tell that a quantity is greater than, less than, or equal to another quantity using various strategies and language. This begins with perceptual cues (which group “looks” bigger) and matching strategies (pairing objects from separate groups). Later, students use their knowledge of counting and cardinality to compare numbers and quantities.

Ordering Numbers and Quantities

Students can put numbers or quantities in the correct order numerically both orally and in written form. This requires an understanding of hierarchical inclusion: the idea that each number (i.e. 8) is exactly 1 greater than the previous number (i.e. 7) and includes all of the numbers that came before it.

Algebraic Thinking and Operations

Algebraic thinking is the process of recognizing, identifying, and analyzing mathematical patterns and generalizing mathematical knowledge to multiple situations. Operations such as addition, subtraction, multiplication, and division are used to reason and solve for unknowns.

Understanding Equations

In mathematics, an equation can be described as two parts, or expressions, that are equal. Students must understand that in order to be true, both sides of the equation must be equal, or the same. Although unknown values traditionally come after the equal sign, students should be able to solve for a missing part at any place in the equation.

To accurately create and solve equations, students must have an understanding of signs (such as = and +), vocabulary, and various strategies.

Operations and Their Properties

Addition

Addition describes the process of adding quantities together to find a sum. Being able to add is directly connected to the ability to count and demonstrate one-to-one correspondence.

Students should be comfortable using vocabulary like addend, sum, total, and plus. They should understand the following signs: + and =. Students begin learning addition through various strategies and eventually remember basic facts through rote memorization.

Subtraction

Subtraction problems involve taking away or comparing numbers. Students should understand that subtraction is the inverse of addition, and that subtraction problems can also be unknown addend problems.

Students should be comfortable using vocabulary like subtract, minus, less, compare, difference, and take away. They should understand and use the following signs: - and =. Like addition, students begin learning subtraction by using various strategies and eventually rote memorization.

Multiplication

Multiplication is the process of adding a number to itself a specific number of times and can also be characterized as repeated addition. For example, 8 x 4 is the same as 8 + 8 + 8 + 8. Students begin to understand the process of multiplication by identifying and adding equal groups and eventually remember basic facts through rote memorization.

Multiplication can also be shown visually through arrays, which are pictures that align items in rows and columns. The multiplication fact 8 x 4 can be represented by items in 8 rows of 4 columns, as shown here:

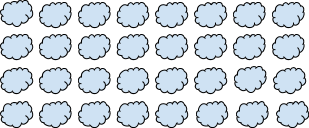
Students should be comfortable using vocabulary like multiply, group, by, and product. They should understand the sign x.

Division

Division is the process of splitting a quantity into equal groups, and is the inverse of multiplication. For example, \(12 \div 3\)could be read as “Divide 12 into 3 equal groups. How many are in each group?”

Because multiplication and division are related, division can also be represented by arrays. Students should be comfortable using vocabulary like divide, quotient, and goes into. They should understand the sign \(\div\).

Properties of Addition and Multiplication

• The commutative property says that changing the order of numbers being added or multiplied does not change the sum. For example:

\[a+b=b+a\] \[a \times b = b \times a\]

• The associative property says that you can add or multiply in any order, regardless of how numbers are grouped by parenthesis. For example:

  • \(a+(b+c)\) is the same as \((a+b)+c\)

  • In multiplication, \(a \times (b \times c)\)is the same as \((a \times b) \times c\).

• The identity property says that for addition, any number + 0 is equal to that number \((a+0=a)\)and for multiplication, any number \(\times\)1 is equal to that number \((a \times 1=a)\)

• The zero property (multiplication only) says that the product of any number and zero is zero \((a \times 0 = 0)\).

• The distributive property (multiplication only) is used when there are parentheses within a problem, like this : \(a(b+c)\)= ____. Usually, according to order of operations, students should complete the problem inside the parentheses first. Sometimes, however, the values inside the parenthesis cannot be added together. In that case, the distributive property tells that multiplying a sum of the numbers in parentheses by a number is the same as multiplying the number by each addend and then adding them together. For example:

\[a(b+c)=ab+ac\]

Solving Problems

As students gain an understanding of addition, subtraction, multiplication, and division, they start to solve problems with two or more numbers. Often, more than one operation is required to solve a problem. In this case, students must use order of operations.

Representing Information in an Equation

An equation shows a relationship between the numbers and symbols on both sides of the equal sign. When there is an unknown, students use various operations (addition, subtraction, multiplication, and division) and strategies to solve and find the unknown.

Solving Equations with Addition and Subtraction

Students should be able to use a variety of strategies to add and subtract numbers, starting with numbers \(0-20\) and gradually generalizing those strategies to add and subtract larger numbers. A strong understanding and practice of these strategies build the foundation for multiplication and division. A few strategies are:

Using Manipulatives

Students can use manipulatives like counters or beads to add by counting out two groups, combining them, counting them, and finding the total. With bigger numbers, students should use manipulatives that represent groups of 10s and 100s like base ten cubes.

Draw a Picture

Students use a drawing tool to draw a picture that represents the problem. Like the use of manipulatives, they can draw, combine, and count to find the total.

Number Line

The use of a number line helps students visualize a problem in a linear and measurable form. Students can compose and decompose numbers and see how numbers are related in terms of their distance from each other. Students “hop” forward or backward on the line in predetermined increments to solve addition and subtraction problems.

Order of Operations

When more than one operation is needed to solve an equation, a specific order is necessary. Often, the acronym PEMDAS is used by students to remember this order.

Expressions within Parentheses are solved first.

• Any Exponents are solved second.
• Multiply and Divide from left to right.
• Add and Subtract from left to right.

Working with Other Math Notations

Students must be able to write, interpret, and evaluate these in order to demonstrate proficiency.

Expressions

An expression is a group of numbers, variables, and signs grouped to represent a quantity. Some examples of expressions are:

\[5+8\] \[9-x\] \[6(x+4)\] \[18 \times 3\]

Notice that while an expression can be part of an equation with an equal sign, an expression does not have an equal sign.

Patterns

In math, a pattern is a sequence that repeats based on a rule. Students begin to identify short, simple patterns with things like colors and shapes, and later evaluate patterns that are longer or involve numbers and equations.

The ability to understand and extend patterns of all kinds is crucial in mathematics. It allows students to begin to reason mathematically and draw connections. Ultimately, students can recognize, identify, and use repeated actions in counting and operations.

Relationships

A relation shows the relationship between two sets of values in an ordered set. The relationship occurs between \(x\) values, which are called the domain, and \(y\) values, which are called the range. Relations are usually displayed as a graph, table, or map.